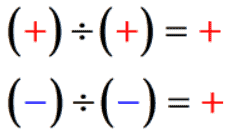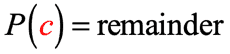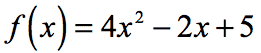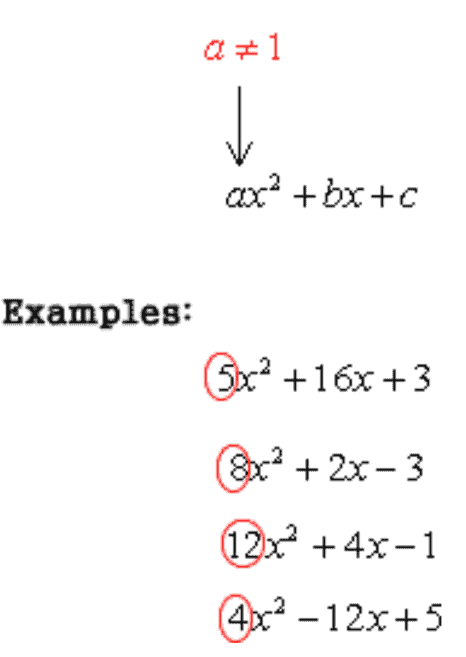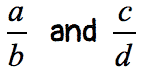El Prueba de raíces racionales (also known as Rational Zeros Theorem) allows us to find all possible rational roots of a polynomial. Suppose a is root of the polynomial Pleft( x right) that means Pleft( a right) = 0. In other words, if we substitute a into the polynomial Pleft( x right) and get zero, 0, it means that the input value is a raíz de la función.
But how do we find the possible list of rational roots? Here’s how it works in a nutshell!
Key Ideas of Rational Roots Test
Suppose we have some polynomial Pleft( x right) with integer coefficients and a nonzero constant term:
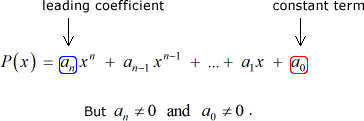
Then every rational root of Pleft( x right) is of the form:
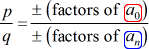
The best way to learn this method is to take a look at some examples!
Examples of How to Find the Rational Roots of a Polynomial using the Rational Roots Test
Ejemplo 1: Find the rational roots of the polynomial below using the Rational Roots Test.
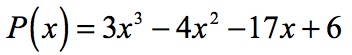
Finding the rational roots (also known as rational zeroes) of a polynomial is the same as finding the rational x-intercepts.
- Start by identifying the constant term a0 and the leading coefficient an.
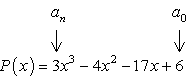
- Determine the positive and negative factors of each.
Factors of constant term, {a_0} = 6,,:,, pm ,left( {1,2,3,6} right)
Factors of leading term, {a_n} = 3,,:,, pm ,left( {1,3} right)
- Write down the list of the possible rational roots by finding {p over q} which is simply the proporción of the factors of the término constante y término principal. Make sure that you keep track of the possible combinations.
This is how I do it. I take each numerator and divide it by all denominators. Then I move on to the next numerator and again divide by all denominators. I keep repeating this process until I have gone through all the numerators. This ensures that we have covered all possible combinations.
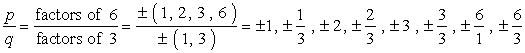
BIG Caution: After you write down all combinations, simplificar the fractions in order to get rid of duplicates.
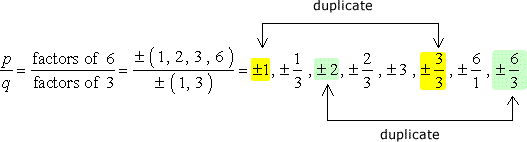
So these are the numbers without duplicates that we will check as possible roots. We have twelve (12) possible candidates to check.
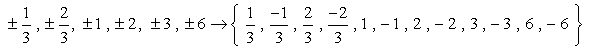
- Remember that if a is a root of the polynomial Pleft( x right), then Pleft( a right) = 0. Now, let’s check each number.
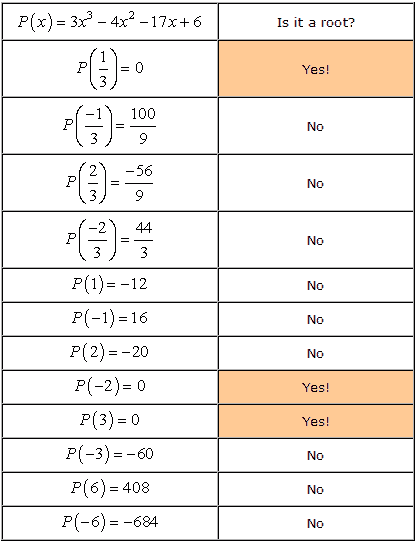
- Therefore, the rational roots of the polynomial
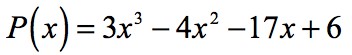
Médica
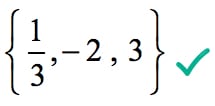
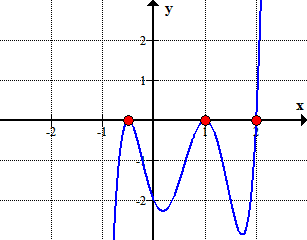
Here is the graph of the polynomial showing where it crosses or touches the x-axis. These are in fact the x-intercepts of the polynomial.
Ejemplo 2: Find the rational roots of the polynomial below using Rational Roots Test.
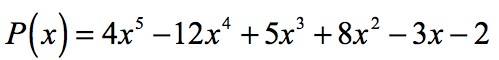
The constant term is a0 = –2 and its possible factors are p = ± 1, ± 2. For the leading coefficient, we have an = 4 and its factors are q = ± 1, ± 2, ± 4.
- To find the possible roots of the polynomial, write in the form

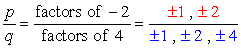
Write down all possible

combinaciones:
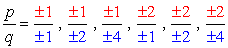
- Simplify each fraction to eliminate duplicates or identical values. Here’s our new and improved list!
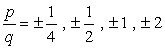
- Due to the plus or minus consideration of each number, we will have eight (8) possible candidates as the roots of this polynomial.
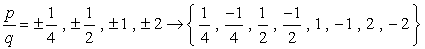
If you plug in each value to the given polynomial and gets zero, that means the number you substituted is a root! Try this on paper, and you should be convinced that there are only three values satisfying this condition.
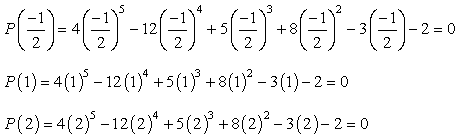
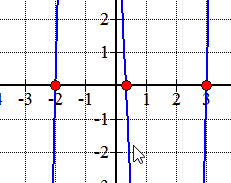
Therefore, the rational roots of the polynomial
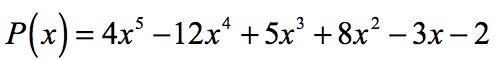
Médica
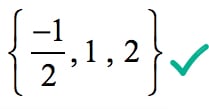
Graphically, it shows that the polynomial touches or crosses the x-axis at those roots determined by rational roots test.